New Technology for a New Century
International Conference 
FIG Working Week 2001, Seoul, Korea 6–11 May 2001

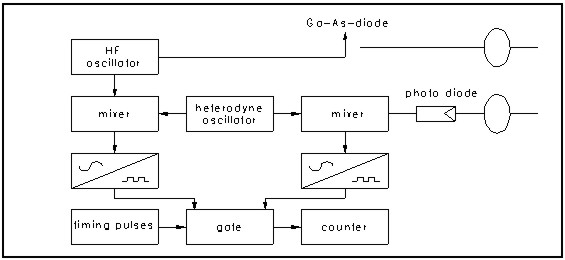
Session 17 - The Use of Positioning and Measurement Technology in Engineering and Construction Applications

Key words: Total station, Indirect leveling method, Incidence angle, Regression analysis.

Total Station are spread to many measuring sites, however, their functions are not fully understood yet. Leveling is one of the misunderstandings. The indirect leveling method using total station for leveling is considered to have due accuracy, applications of the indirect leveling is gradually expanding for public works such as construction of roads, airports and cities.

Instead of direct leveling method, indirect leveling method using total station is applied in this research to assure the technicians in performing the tests, it is more economical as well.

The results are expected to be used for many public works including routine survey, wide residential land development and subsidence measuring instruments.

At present, Total Station has been widely spread and used in many survey sites, and sometimes it is not fully used since users misunderstand the principles of this unit. One of them is the leveling, and in case we use Total Station for leveling, this is classified as the indirect leveling method, and since it is judged that this method can maintain the considerable accuracy, now it has been increasingly used for many public works such as road, airport and city etc.

This study empirically made a research in the improvement of accuracy of precise leveling by using the indirect leveling method, Total Station that can more simply and quickly find elevation by replacing the direct leveling.

The principle of the measurement device, EDM, which is currently used, is that it calculates the distance by measuring the phase shift during the radiated light wave from EDM's main unit returns by being reflected through the reflector, which is positioned at measurement point. This phase shift can be regarded as a part of frequency that appears as the unit of time or length under a specific condition.

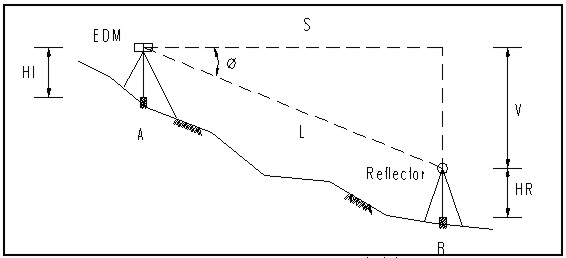
Figure 1. EDM's structure

When the slope distance L and slope angle is measured by EDM, if the elevation of point A is the reference point, we can find the elevation of point B by the following formula(2-1).

Elevation of Point B = Elevation of Point A + HI±L sin - HR (2-1)

Figure 2. EDM's measurement principle

The distance measured by EDM is expressed as the formula (2-2).

        (2-2)

Here, we have : Phase shift of the reflected light wave

: Wavelength

: Number of transmitted wavelength

If we measure repeatedly 2 or 3 respectively different wavelengths in order to check Value , we can know Wavelength is the function of Frequency and Electric wave’s velocity .

                              (2-3)  

Though electronic wave's velocity is 299792.5km/sec the same as light velocity under vacuum, but since it always slower than light velocity in the atmosphere, we can correct the influence by the atmosphere and calculate it by the following formula.

                                 (2-4)

Here, we have : air's refractive index.

We should measure the temperature and humidity in the air according to measurement line if we try to find the exact Value .

If we substitute formula (2-4) for formula (2-3), the Value of the transmitted signal becomes,

                                (2-5)

and if we assume the wavelength under a specific atmosphere condition is , since it is described as

                            (2-6)

we can express EDM's distance is , and can be expressed by the phase shift of .

If it is during the measurement, the corrected value of is,

                           (2-7)

and at this time, the real distance is

                   (2-8)

From the formula (2-7) and formula (2-8), we can get

                           (2-9)

and the corrected distance, that is, the correction formula of the measurement distance under a specific atmosphere condition is expressed as the following formula (2-10).

        (2-10)

In order to find the final corrected distance, we should correct the error of EDM's zero point and add Earth curvature, slope and the corrected error of average sea level , and consequently the formula for the final corrected distance is as shown in the following formula (2-11).

                 (2-11)

Here, we have : Measured distance

: Refractive index when correcting in Lab

: Refractive index at the moment of measuring

If we substitute the formula (2-6) and (2-10) for (2-11), the corrected distance

is as follows.

     (2-12)

The variance of the distance is as follows.

(2-13)

And if we simply express the formula (2-13) by , it is described as follows.

                             (2-14)

Here, we have : , the standard deviation of the total value

EDM's accuracy is expressed as the following general formula.

or                             (2-15)

And if we express the formula (2-14) by the form of formula (2-15), , is expressed as follows.

                                                                         (2-16)

                                               (2-17)

Here, we have

: Error of electric wave's velocity same as light velocity under vacuum
: Error of modulated frequency
: Error of refraction coefficient
: Error of phase shift measurement
: Error of zero point
: Geometric error not included in the formula (2-15)

The device for measuring distance by light wave always should have the correction for the measured value. Every kind of distance measurement device is the same, but they are generally influenced by the following factors. Since the weather condition already exists, the difference of influence made by measurement devices is combined with the observation variable that we can directly observe and the non-observation variable that we can not directly observe.

The variables that are possible to observe and determine a specific condition are temperature, atmospheric pressure and steam pressure, and the effect of them is various. The refraction index is calculated by the variables of temperature, atmospheric pressure and steam pressure, and it determines the ratio between the velocities of electromagnetic wave's electric wave under vacuum () and electric wave under the regular atmospheric condition (). Therefore, it is expressed as , and if we know the refraction index, which is always less than 1, we can know the temporary spread velocity of electromagnetic wave and this equals to the spread velocity of measurement signal.

Though the measurement signal progresses the same distance with the various velocities according to the weather condition, since the computer installed at the measurement device is programmed in advance to use a certain fixed wave velocity, this velocity deviation comes to generate the signals in proportion to the measured distance.

                           (3-1)

Here, we have

: Temperature (℃)
: Pressure ()
: Refraction index to the wavelength of the reflected frequency under ℃ and
760
: 0.003661(1/℃), Expansion coefficient of air per 1℃

The variables that are impossible to directly observe and influence on the measurement process are rapid weather change, rainfall, heat haze and fog etc. Therefore it is desirable necessarily to correct the factors that largely influence on the measurement result, as much as possible. The variables to be corrected are refraction of atmosphere, height of sea level, refraction by projection method and difference of scale coefficient and so on.

              (3-2)

Here, we have

: Horizontal distance
: Difference of elevation
: Slope distance
: Ceiling angle
: Earth Refraction index
: Influence by refraction
: Height of horizontal, central axis of device reference point
: Earth radius (6,377km)

The distance correction value, in sea level is as follows.

                                                       (3-3)

Here, we have

: Horizontal distance from sea level to reflector's height
: Reflector's height in sea level

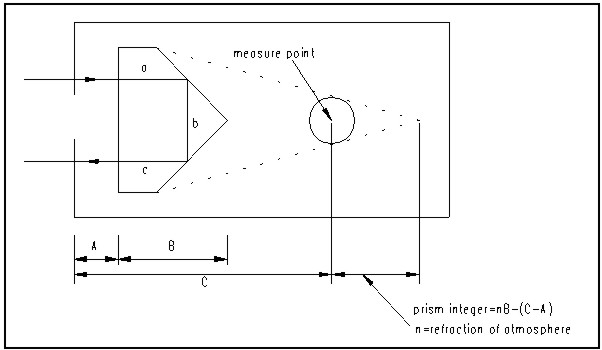
3.2.1. Installation of reflector

Since a prism reflector decreases if its slope angle is getting bigger to prism face, and it has much dispersion of light if measurement distance is getting longer, we should install a reflector uprightly facing to a measurement device when we install it.

(1) Prism integer: The light radiated from the distance measurement device by light wave is reflected through the inside of prism, and if we convert the inside length into the distance in the air, it becomes longer two times.

It is shown in Fig. 3 as follows.

Since a prism has its own integer, it is better to use a prism that exactly fits to a distance measurement device by light wave, and if we use it under the condition that a prism's integer is wrong or it is not exactly adjusted, we may get large measurement error. In Fig. 3, the distance we actually measure is Line , but what we try to find is Line . The difference between Line B and Line becomes the prism's integer.

Since the refraction index of prism is higher than the atmosphere, we should consider this refraction index and decide it, and generally the prism's integer is expressed as follows.

                 (3-4)

Here, we have

: Refraction index of the atmosphere
: B in Fig. 3
: in Fig. 3

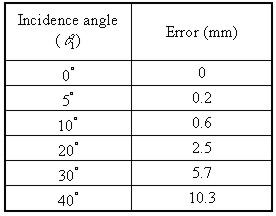
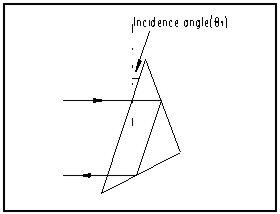
Figure 3 Prism integer

(2) Error by incidence angle

Since the error happens according to the light angle that enters into a prism, it is desirable to set up a prism side vertically and exactly to the measuring point when we set up a prism.

The following Table 1 shows the experimental value that TOPCON Co. executed at 1km section by prism's incidence angle.

Table 1 Distance error volume by

 Figure 4. Distance error by Incidence angle

We selected the asphalt-paved road equivalent to total 650m near Namchun-Dong, Sooyoung-Ku, Pusan, Korea as the measurement object area. We used TOPCON's GTS-701, Total Station as the measurement device, and in order for us to compare the accuracy, we also used TOPCON's first class leveling device as the leveling device, and the specifications for each device is as shown in the following Table 2

Table 2 measurement device

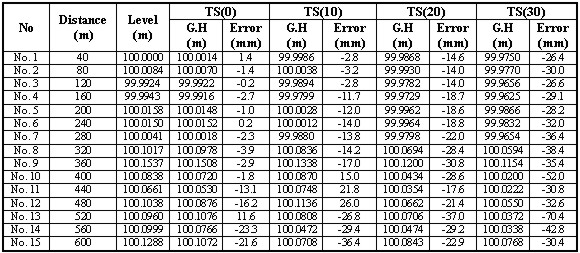
We selected a random point (No. 0) in the measurement object area, and we set out total 15 measuring points at the same 40m intervals from that point as reference. The distance between a target staff and a device is decided by several conditions, and since the collimation distance of leveling that requires high precision is 40m, this study also set the same interval of the target staff as 40m and measured each measuring point by the first class leveling device. And when we measured it by Total Station, we fixed the device at the reference point(No. 0), and made the heights of the device and reflector same, and we selected the direction of the incidence angle that faced downward, and we measured and compared it with the value that was measured by the first class leveling device and analyzed it.

The result that we measured respectively three times by the first class leveling device and Total Station and calculated the average value is as shown in Table 3 and Fig. 5. As a measurement result, it showed that the rearranged error to the distance of 600m measured by the first class leveling device was 0.6mm, and it satisfied the allowable error of the first class leveling, 1.9mm. And in case measuring by Total Station, it showed that the maximum distance to discriminate was 280m, and if we see the measured value without a prism's incidence angle, the error was 0.2∼23.3mm.

These are the values appeared by each distance for No. 0 point, and it is considered that they appeared because of the observer's collimation error, light wave's dispersion coming from Total Station's main unit and since it was not vertically positioned to the exact central point of a prism.

And when measuring by Total Station, we could calculate the correlation between the incidence angle and the error volume as the form of

Table 3 Measurement result

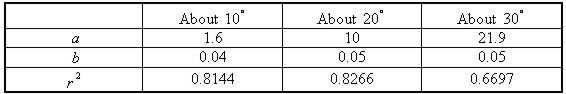
Figure 5 Measurement result

1. As a result that we measured the indirect leveling by Total Station, it was observed that the maximum distance we could discriminate the prism's central point from the telescope lens was 280m, and its error was 2.3mm that satisfies the second class allowable error, 2.6mm. Therefore it is judged that if we apply the distance that can discriminate the prism's central point, it can satisfy the second class leveling.

2. From the result that we made the regression analysis on the correlation of prism's incidence angle when measuring an indirect leveling by Total Station, the following correlation was formed at the distance of 0∼400m.
   y = a + bx  ;  y = error volume (±mm), x = distance(m)

3. It is judged that if we calculate the correction value between the distance and incidence angle when measuring an indirect leveling by Total Station and apply it, we can find the more exact leveling value.

1. Noboru Inouchi, On the Accuracy of Precise Leveling, Geodetic Society of Japan, Vol. 29, No. 2, 1983, pp. 89∼93.

2. kye-Hak, Lee, A Study on the Effects of Refraction in the Precise Leveling, Journal of the Korean Society of Geodesy, Photogrammetry, and Cartography, Vol. 8, No. 3, 1988, p. 81.

3. Tae-suc, Kang, cadastre survey, hyungseul publish, 1994, pp. 229 -243.

4. Michel KASSER, Precision des Mesures Electroniques de Distances et Reflecteurs, FIG, Commission 5, 1994, pp. TS 502/1-502/8.

5. Electronic GTS-701 Total Station Instruction Manual, TOPCON.

6. TS-E1 LEVEL Instruction Manual, TOPCON.

7. Byung Guk, Kim, Study on the Error Estimation in EDM Measurements, Journal of the Korean Society of Geodesy, Photogrammetry, and Cartography, Vol. 18, No. 4, 1998, p. 496-498.

CONTACT

Prof. Jongchool Lee
Dept. of Civil Engineering
Pukyong National University
100 Yongdang Dong
Nam-Gu
Pusan
KOREA
Tel. + 82 51 622 1662
E-mail: jclee@pknu.ac.kr 

Taeho Rho
Ph.D. Course
Dept. of Civil Engineering
Pukyong National University
100 Yongdang Dong
Nam-Gu
Pusan
KOREA
Tel. + 82 51 622 1662
E-mail: rth1348@hanmail.net 

20 April 2001

This page is maintained by the FIG Office. Last revised on 15-03-16.